The Koch snowflake (also known as the Koch star and Koch island) is a mathematical curve and one of the earliest fractal curves to have been described. It is based on the Koch curve, which appeared in a 1904 paper titled "On a continuous curve without tangents, constructible from elementary geometry" (original French title: "Sur une courbe continue sans tangente, obtenue par une construction géométrique élémentaire") by the Swedish mathematician Helge von Koch.
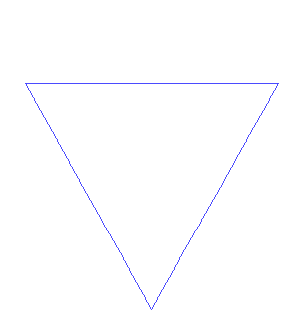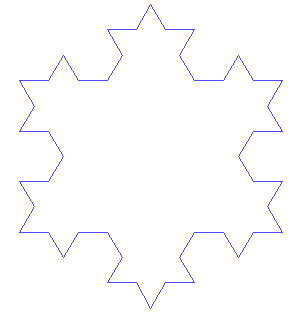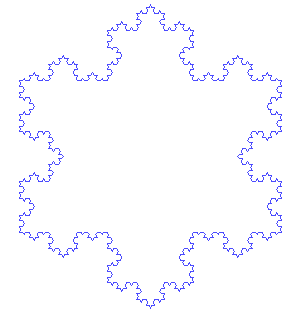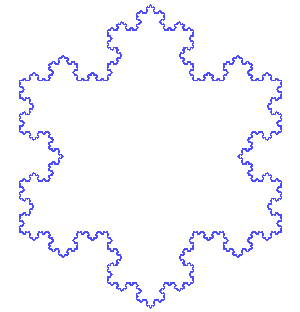
Here are some ascii representations of various iterations:
n=1
__
\/
n=2
__/\__
\ /
/_ _\
\/
n=3
__/\__
\ /
__/\__/ \__/\__
\ /
/_ _\
\ /
__/ \__
\ /
/_ __ __ _\
\/ \ / \/
/_ _\
\/
Since there is obviously a limit to the resolution of the ascii representation, we must enlarge the size of the snowflake by a factor of 3 for each iteration to show the extra detail.
Write the shortest code to output the snowflake in the same style for n=4
Your program should not take any input.
Your program should write the snowflake to the console.
