BBC BASIC
Rev 1 Golfed code, 655 ASCII characters, tokenised filesize 614
Some major improvements to the data table, by hashing the string A.B..N to a number (1*A+2*B+..n*N)+n before looking up, and by storing only one translation vector (the other one is generated by code.) More explanation when I have finished golfing.
t=PI*2DIMm(9)
c=0z=0INPUTz$
FORi=1TOLEN(z$)d%=VAL(MID$(z$,i))IFd%c+=1m(c)=d%i-=d%=12z+=c*d%
NEXTREPEATREADl,e,f
UNTILl=z+c
l=4-3*(m(3)MOD3=0)-8*(l=59)
DATA69,0,70,65,100,35,66,149,0,49,109,0,52,80,0,55,0,189,39,120,0,44,40,40,58,55,95,47,136,0,59,40,0
VDU23,23,3|
FORr=-9TO19FORs=-9TO9a=1+e*(r*2+s)-f*l*s/4b=1+f*(r*2+s)+e*l*s/4p=40q=0FORk=1TOm(c)/2FORj=1TOc
n=m(j)o=TAN(PI/3)IFe=109ANDn<>4o=1
w=-p*COS(t/n)-q*SIN(t/n)q=p*SIN(t/n)-q*COS(t/n)p=w
u=p:v=q
x=a:y=b
MOVEx,y
FORi=1TO14x+=u*2y+=v*2IFVAL(z$)DRAWx,y ELSEGCOL9LINEx-u-v/o,y-v+u/o,x-u+v/TAN(PI/n),y-v-u/TAN(PI/n)
w=v*COS(t/n)-u*SIN(t/n)u=v*SIN(t/n)+u*COS(t/n)v=w
NEXTNEXT
p=u:q=v
a=x:b=y
NEXTNEXTNEXT
Rev 0 Golfed code, 770 ASCII characters, tokenised filesize 728
All I've done here is remove comments, unnecessary whitespace and quote marks, and put all the DATA on one line. There's certainly room for more golfing.
t=PI*2DIMm(9)
c=0INPUTz$
FORi=1TOLEN(z$)d%=VAL(MID$(z$,i))IFd%c+=1:m(c)=d%:i-=d%=12
NEXTREPEATREADl$,e,f,g,h
UNTILMID$(z$,1-(VAL(z$)=0))=l$
DATA3.3.3.3.3.3,240,0,120,70,3.3.3.3.6,200,70,40,210,3.3.3.4.4,80,0,40,150,3.3.4.3.4,-40,150,150,40,3.12.12,300,0,150,260,3.4.6.4,220,0,110,188,3.6.3.6,160,0,80,140,4.4.4.4,80,0,0,80,4.6.12,0,380,330,-190,4.8.8,272,0,136,136,6.6.6,240,0,120,70
VDU23,23,3|
FORr=-9TO19 FORs=0TO9a=1+e*r+g*s
b=1+f*r+h*s
p=40q=0FORk=1TOm(c)/2FORj=1TOc
n=m(j)o=TAN(PI/3):IFe=220ANDn<>4o=1
w=-p*COS(t/n)-q*SIN(t/n)q=p*SIN(t/n)-q*COS(t/n)p=w
u=p:v=q
x=a:y=b
MOVEx,y
FORi=1TO14x+=u*2y+=v*2IFVAL(z$)DRAWx,y ELSEGCOL9LINEx-u-v/o,y-v+u/o,x-u+v/TAN(PI/n),y-v-u/TAN(PI/n)
w=v*COS(t/n)-u*SIN(t/n)u=v*SIN(t/n)+u*COS(t/n)v=w
NEXTNEXT
p=u:q=v
a=x:b=y
NEXTNEXTNEXT
Explanation
This is a continuation of my previous Level 1 answer, but I decided to post it separately because it's rather long.
Level 2
This is achieved by translation of my "level 1.5" templates from my previous answer. The two translation vectors for each tiling are hardcoded. I take advantage of the fact that an isosceles triangle of base 80 and height 70 is a very good approximation of an equilateral triangle, and a right triangle with hypotenuse vector (56,56) has a hypotenuse length very close to 80.
Level 3
To plot the duals, instead of plotting an edge of the polygon, we plot a spoke from the middle of that edge to the centre of the polygon. This is at right angles to the edge and has length of 1/TAN/(PI/n) times of the vector (u,v) which in turn is half as long as the edge.
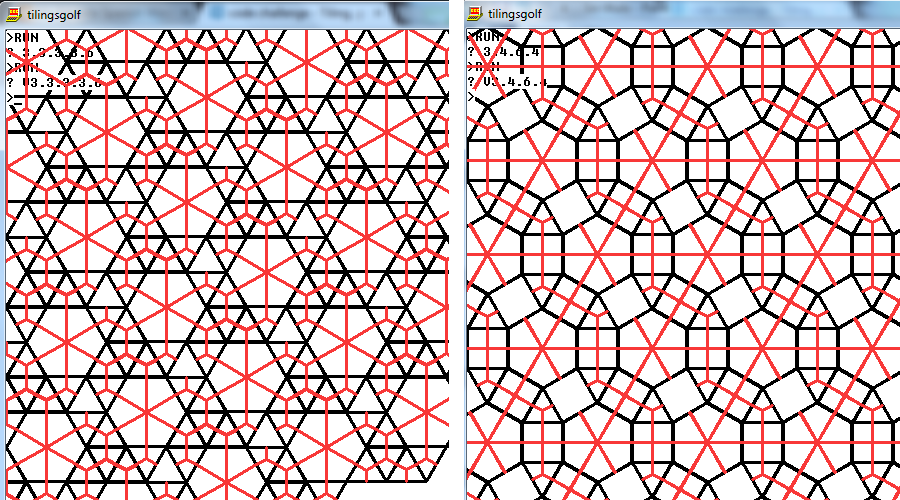
Unfortunately, because certain polygons in tilings 3.3.3.3.6 and 3.4.6.4 are not plotted explicitly, they would not be plotted if we only did this. Therefore the spoke also extends outward from the polygon. The outward extension is controlled by the variable o.
By default the extension is sufficient to reach the centre of a triangle, but for 3.4.6.4 it needs to be extended more in order to draw the duals of the squares that are not plotted explicitly. So enough extension to fill in the missing squares is applied when hexagons and triangles are plotted explicitly, but the normal extension is applied when squares are plotted explicitly, to avoid spurious lines in the adjacent triangles.
Here's what they look like without the spoke extensions. The holes in the dual pattern can be clearly seen. The correct output can be seen in the main picture at the bottom of the answer
Commented Code
Differences from my previous answer are indicated inline
t=PI*2 :REM constant Tau = PI*2
DIMm(9) :REM declare array for the numbers in the input
c=0 :REM number of polygons in the list
INPUTz$
FORi=1TOLEN(z$) :REM for each character in the input
d%=VAL(MID$(z$,i)) :REM use VAL to return the numeric value of the substring to the right and store to integer variable
IF d% c+=1 :m(c)=d%: i-=d%=12 :REM if the last character read was a number, d% contains it, otherwise 0. Advance c and store to m. If it is 12, increment i to skip a character.
NEXT
REM BLOCK OF NEW CODE to define vectors (e,f) and (g,h) for each possible tiling
REPEAT
READ l$,e,f,g,h :REM read an entire line of the data below
UNTIL MID$(z$,1-(VAL(z$)=0))=l$ :REM abort the loop when l$ coincides with the input. the MID$ strips off the 'V' from the input where necessary.
DATA"3.3.3.3.3.3",240,0,120,70
DATA"3.3.3.3.6",200,70,40,210
DATA"3.3.3.4.4",80,0,40,150
DATA"3.3.4.3.4",-40,150,150,40
DATA"3.12.12",300,0,150,260
DATA"3.4.6.4",220,0,110,188
DATA"3.6.3.6",160,0,80,140
DATA"4.4.4.4",80,0,0,80
DATA"4.6.12",0,380,330,-190
DATA"4.8.8",272,0,136,136
DATA"6.6.6",240,0,120,70
VDU23,23,3| :REM change linewidth to 3 (default is 1)
REM END BLOCK OF NEW CODE
FORr=-9TO19 FORs=0TO9 :REM two new loops for translations
a=1+e*r+g*s :REM modified code for
b=1+f*r+h*s :REM coordinates to start drawing at
p=40:q=0 :REM vector of first line
FORk=1TOm(c)/2 :REM draw half as many vertex figures as there are sides on the last polygon in the list
FORj=1TOc :REM for each polygon on the list
n=m(j) :REM n=number of sides
o=TAN(PI/3): IF e=220 AND n<>4 o=1 :REM new code for the spoke extension 1/o.
w=-p*COS(t/n)-q*SIN(t/n) :REM rotate the starting vector anticlockwise by the internal angle of the current polygon
q=p*SIN(t/n)-q*COS(t/n) :REM to avoid overlapping the previous one, if any.
p=w
u=p:v=q :REM make a local copy of the vector and coordinates
x=a:y=b :REM to avoid corruption of p,q,a,b during the drawing of the polygon
MOVE x,y :REM move the graphics cursor to the start without drawing
FORi=1TO14 :REM do 14 iterations regardless of the number of sides on the polygon
x+=u*2 :REM increment x and y by the vector representing the side
y+=v*2 :REM the value is double (u,v) to facilitate drawing duals later
REM if z$ begins with a numeric character, draw an edge. If not, change to red and draw a spoke.
IFVAL(z$) DRAW x,y ELSE GCOL9: LINEx-u-v/o,y-v+u/o,x-u+v/TAN(PI/n),y-v-u/TAN(PI/n)
w=v*COS(t/n)-u*SIN(t/n) :REM rotate the vector clockwise
u=v*SIN(t/n)+u*COS(t/n) :REM through the external angle of the polygon
v=w
NEXT :REM draw next edge of the current polygon
NEXT :REM draw next polygon of the current vertex
p=u:q=v :REM once the vertex is finished, we will be two sides around the perimeter of the last polygon.
a=x:b=y :REM copy the position and direction data into p,q,a,b.
NEXT :REM draw next vertex figure
NEXT :REM close the two new translation loops
NEXT
Output
The program performs only one tiling or dual for each run. However it plots the duals in red. To save space, I ran the program twice without clearing the screen in order to superimpose the dual on top of the regular tiling.
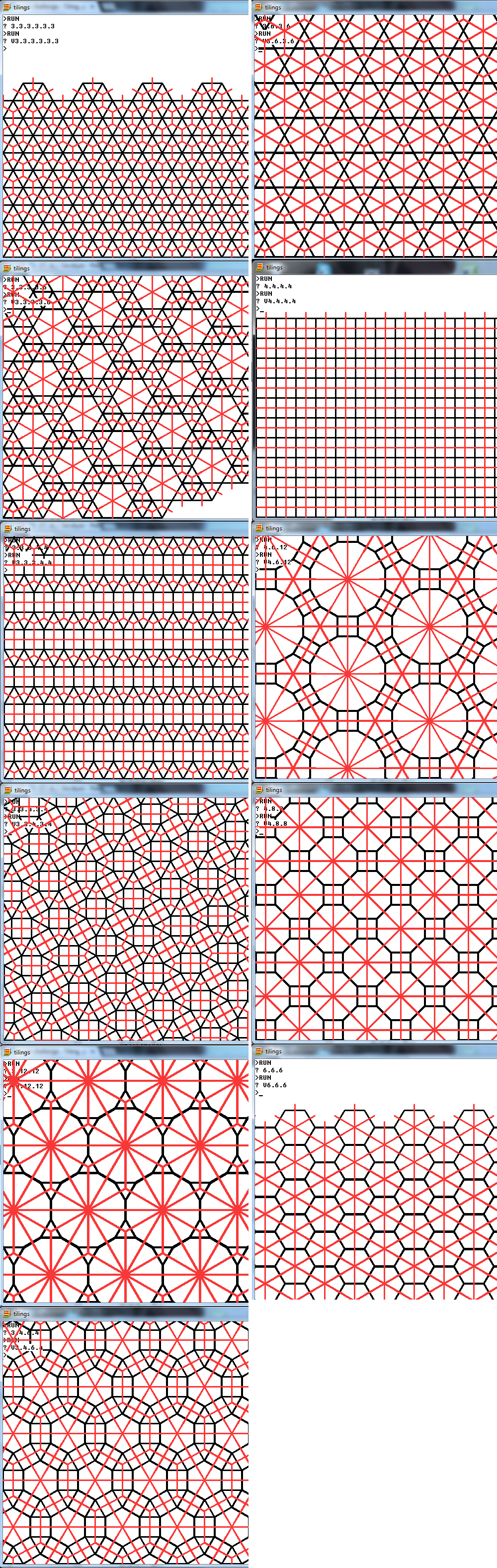





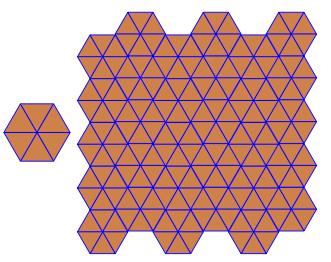
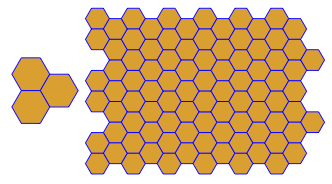
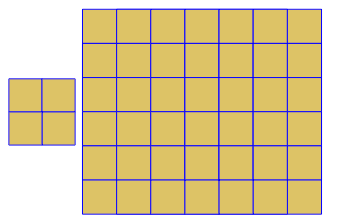
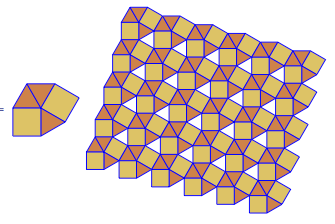
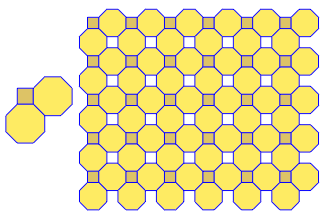
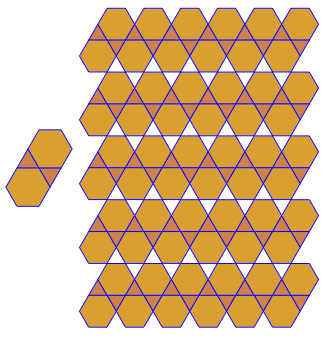

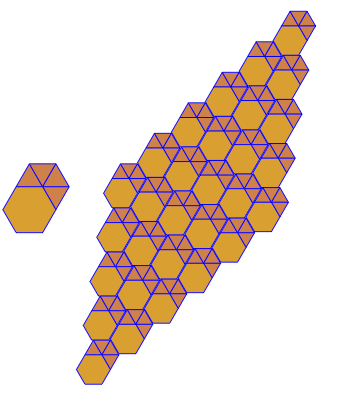
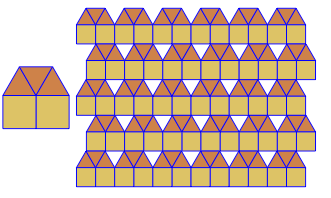
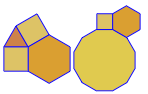
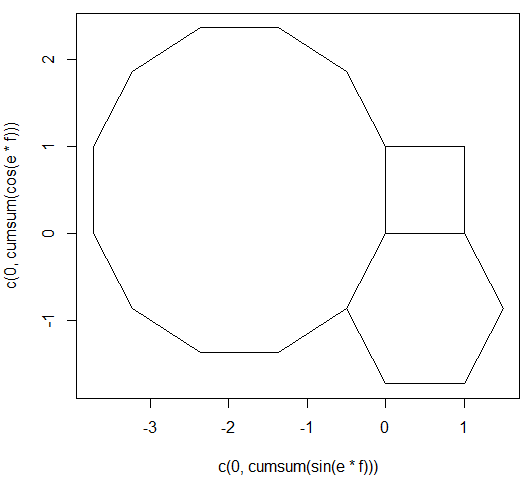
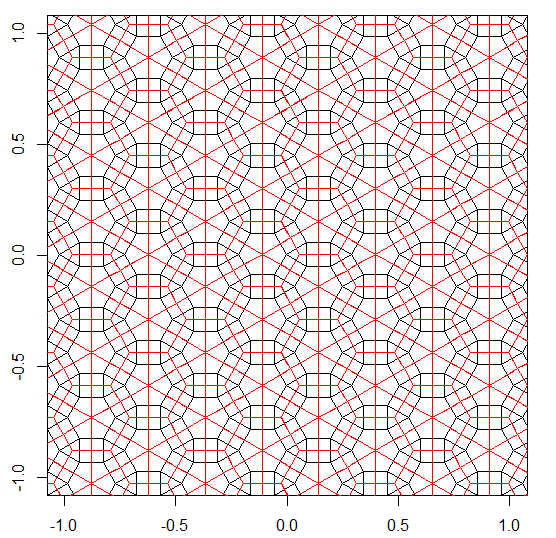

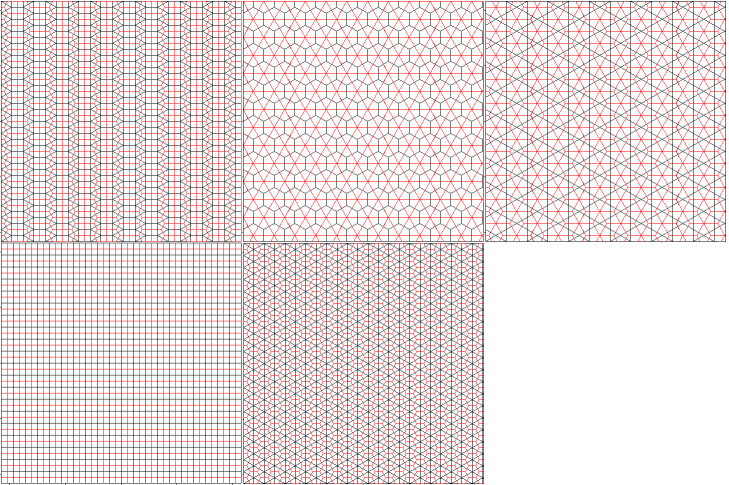

3.3.3.4.43.3.4.4.33.4.4.3.34.4.3.3.34.3.3.3.4. Do we have to support all synonyms, or just the lexically lowest one (as given in the question)? Also,3.3.3.3.6exists in two mirror image forms. I understand either is acceptable. \$\endgroup\$3.3.3.4.4is missing for example. en.wikipedia.org/wiki/… matches your list exactly. I understand outline or filled polygons are acceptable (or a combination of the two?) Some duals are already in the list. For example4.4.4.4is its own dual and3.3.3.3.3.3and6.6.6are mutally dual. As the duals are displayed independently of their parents, I understand there is no need for correct alignment with the parent. \$\endgroup\$3.3.3.3.6but how would you know which one it is? :) \$\endgroup\$