Sylvester's sequence

Question

Sylvester's sequence, OEIS A000058, is an integer sequence defined as follows:

Each member is the product of all previous members plus one. The first member of the sequence is 2.

Task

Create a program to calculate the nth term of Sylvester's Sequence. Standard input, output and loopholes apply.

Standard sequence rules apply.

This is code-golf, so the goal is to minimize the size of your source code as measured in bytes.

Test Cases

You may use either zero or one indexing. (Here I use zero indexing)

>>0
2
>>1
3
>>2
7
>>3
43
>>4
1807

Answer 1

Brain-Flak, 76 68 58 52 46 bytes

<>(()())<>{({}[()])<>({({}[()])({})}{}())<>}<>

Try it online!

Uses this relationship instead:

$$a(n) = 1+\sum^{a(n-1)-1}_{i=0} 2i$$

which is derived from this relationship modified from that provided in the sequence:

$$a(n+1) = a(n)(a(n) - 1) + 1.$$

Explanation

For a documentation of what each command does, please visit the GitHub page.

There are two stacks in Brain-Flak, which I shall name as Stack 1 and Stack 2 respectively.

The input is stored in Stack 1.

<>(()())<>             Store 2 in Stack 2.

{                      while(Stack_1 != 0){
  ({}[()])                 Stack_1 <- Stack_1 - 1;
  <>                       Switch stack.
  ({({}[()])({})}{}())     Generate the next number in Stack 2.
  <>                       Switch back to Stack 1.
}

<>                     Switch to Stack 2, implicitly print.

For the generation algorithm:

({({}[()])({})}{}())      Top <- (Top + Top + (Top-1) + (Top-1) + ... + 0) + 1

(                  )      Push the sum of the numbers evaluated in the process:

 {            }               while(Top != 0){
  ({}[()])                        Pop Top, push Top-1    (added to sum)
          ({})                    Pop again, push again  (added to sum)
                              }

               {}             Top of stack is now zero, pop it.
                 ()           Evaluates to 1 (added to sum).

Alternative 46-byte version

This uses only one stack.

({}<(()())>){({}<({({}[()])({})}{}())>[()])}{}

Try it online!

Answer 2

Jelly, 5 bytes

Ḷ߀P‘

This uses 0-based indexing and the definition from the challenge spec.

Try it online! or verify all test cases.

How it works

Ḷ߀P‘  Main link. Argument: n

Ḷ      Unlength; yield [0, ..., n - 1].
 ߀    Recursively apply the main link to each integer in that range.
   P   Take the product. This yields 1 for an empty range.
    ‘  Increment.

Answer 3

Hexagony, 27 bytes

1{?)=}&~".>")!@(</=+={"/>}*

Unfolded:

    1 { ? )
   = } & ~ "
  . > " ) ! @
 ( < / = + = {
  " / > } * .
   . . . . .
    . . . .

Try it online!

Explanation

Let's consider the sequence b(a) = a(n) - 1 and do a little rearranging:

b(a) = a(n) - 1
     = a(n-1)*(a(n-1)-1) + 1 - 1
     = (b(n-1) + 1)*(b(n-1) + 1 - 1)
     = (b(n-1) + 1)*b(n-1)
     = b(n-1)^2 + b(n-1)

This sequence is very similar but we can defer the increment to the very end, which happens to save a byte in this program.

So here is the annotated source code:

^{Created with Timwi's HexagonyColorer.}

And here is a memory diagram (the red triangle shows the memory pointer's initial position and orientation):

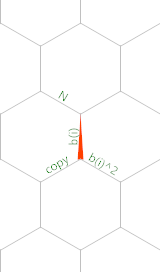
^{Created with Timwi's EsotericIDE.}

The code begins on the grey path which wraps the left corner, so the initial linear bit is the following:

1{?)(
1      Set edge b(1) to 1.
 {     Move MP to edge N.
  ?    Read input into edge N.
   )(  Increment, decrement (no-op).

Then the code hits the < which is a branch and indicates the start (and end) of the main loop. As long as the N edge has a positive value, the green path will be executed. That path wraps around the grid a few times, but it's actually entirely linear:

""~&}=.*}=+={....(

The . are no-ops, so the actual code is:

""~&}=*}=+={(
""             Move the MP to edge "copy".
  ~            Negate. This is to ensure that the value is negative so that &...
   &           ...copies the left-hand neighbour, i.e. b(i).
    }=         Move the MP to edge b(i)^2 and turn it around.
      *        Multiply the two copies of b(i) to compute b(i)^2.
       }=      Move the MP back to edge b(i) and turn it around.
         +     Add the values in edges "copy" and b(i)^2 to compute
               b(i) + b(i)^2 = b(i+1).
          ={   Turn the memory pointer around and move to edge N.
            (  Decrement.

Once this decrementing reduces N to 0, the red path is executed:

")!@
"     Move MP back to edge b(i) (which now holds b(N)).
 )    Increment to get a(N).
  !   Print as integer.
   @  Terminate the program.

Answer 4

J, 18 14 12 bytes

This version thanks to randomra. I'll try to write a detailed explanation later.

0&(]*:-<:)2:

J, 14 bytes

This version thanks to miles. Used the power adverb ^: instead of an agenda as below. More explanation to come.

2(]*:-<:)^:[~]

J, 18 bytes

2:`(1+*/@$:@i.)@.*

0-indexed.

Examples

   e =: 2:`(1+*/@$:@i.)@.*
   e 1
3
   e 2
7
   e 3
43
   e 4
1807
   x: e i. 10
2 3 7 43 1807 3263443 10650056950807 113423713055421862298779648 12864938683278674737956996400574416174101565840293888 1655066473245199944217466828172807675196959605278049661438916426914992848    91480678309535880456026315554816
   |: ,: x: e i. 10
                                                                                                        2
                                                                                                        3
                                                                                                        7
                                                                                                       43
                                                                                                     1807
                                                                                                  3263443
                                                                                           10650056950807
                                                                              113423713055421862298779648
                                                    12864938683278674737956996400574416174101565840293888
165506647324519994421746682817280767519695960527804966143891642691499284891480678309535880456026315554816

Explanation

This is an agenda that looks like this:

           ┌─ 2:
           │    ┌─ 1
       ┌───┤    ├─ +
       │   └────┤           ┌─ / ─── *
── @. ─┤        │     ┌─ @ ─┴─ $:
       │        └─ @ ─┴─ i.
       └─ *

(Generated using (9!:7)'┌┬┐├┼┤└┴┘│─' then 5!:4<'e')

Decomposing:

       ┌─ ...
       │
── @. ─┤
       │
       └─ *

Using the top branch as a the gerund G, and the bottom as the selector F, this is:

e n     <=>     ((F n) { G) n

This uses the constant function 2: when 0 = * n, that is, when the sign is zero (thus n is zero). Otherwise, we use this fork:

  ┌─ 1
  ├─ +
──┤           ┌─ / ─── *
  │     ┌─ @ ─┴─ $:
  └─ @ ─┴─ i.

Which is one plus the following atop series:

            ┌─ / ─── *
      ┌─ @ ─┴─ $:
── @ ─┴─ i.

Decomposing further, this is product (*/) over self-reference ($:) over range (i.).

Answer 5

Perl 6, 24 bytes

{(2,{1+[*] @_}...*)[$_]}

{(2,{1+.²-$_}...*)[$_]}

Explanation

# bare block with implicit parameter ｢$_｣
{
  (
    # You can replace 2 with 1 here
    # so that it uses 1 based indexing
    # rather than 0 based
    2,

    # bare block with implicit parameter ｢@_｣
    {
      1 +

      # reduce the input of this inner block with ｢&infix:<*>｣
      # ( the input is all of them generated when using a slurpy @ var )
      [*] @_

      # that is the same as:
      # ｢@_.reduce: &infix:<*>｣
    }

    # keep calling that to generate more values until:
    ...

    # forever
    *

  # get the value as indexed by the input
  )[ $_ ]
}

Usage:

my &code = {(2,{1+[*] @_}...*)[$_]}

say code 0; # 2
say code 1; # 3
say code 2; # 7
say code 3; # 43
say code 4; # 1807

# you can even give it a range
say code 4..7;
# (1807 3263443 10650056950807 113423713055421844361000443)

say code 8;
# 12864938683278671740537145998360961546653259485195807
say code 9;
# 165506647324519964198468195444439180017513152706377497841851388766535868639572406808911988131737645185443
say code 10;
# 27392450308603031423410234291674686281194364367580914627947367941608692026226993634332118404582438634929548737283992369758487974306317730580753883429460344956410077034761330476016739454649828385541500213920807

my $start = now;
# how many digits are there in the 20th value
say chars code 20;
# 213441

my $finish = now;
# how long did it take to generate the values up to 20
say $finish - $start, ' seconds';
# 49.7069076 seconds

Answer 6

Haskell, 26 bytes

f n|n<1=2|m<-f$n-1=1+m*m-m

Usage example: f 4 -> 1807.

Answer 7

Java 7, 46 42 bytes

int f(int n){return--n<0?2:f(n)*~-f(n)+1;}

Uses 0-indexing with the usual formula. I swapped n*n-n for n*(n-1) though, since Java doesn't have a handy power operator, and the f() calls were getting long.

Answer 8

Haskell, 25 bytes

(iterate(\m->m*m-m+1)2!!)

Answer 9

S.I.L.O.S, 60 bytes

readIO 
p = 2
lblL
r = p
r + 1
p * r
i - 1
if i L
printInt r

Try it online!

Port of my answer in C.

Answer 10

Oasis, 4 bytes

Probably my last language from the golfing family! Non-competing, since the language postdates the challenge.

Code:

²->2

Alternative solution thanks to Zwei:

<*>2

Expanded version:

a(n) = ²->
a(0) = 2

Explanation:

²    # Stack is empty, so calculate a(n - 1) ** 2.
 -   # Subtract, arity 2, so use a(n - 1).
  >  # Increment by 1.

Uses the CP-1252 encoding. Try it online!

Answer 11

Brain-Flak, 158 154 bytes

Leaky Nun has me beat here

({}<(()())>){({}[()]<<>(())<>([]){{}<>({}<<>(({}<>))><>)<>({<({}[()])><>({})<>}{})<>{}([])}{}<>({}())([]){{}({}<>)<>([])}{}<>>)}{}([][()]){{}{}([][()])}{} 

Try it Online!

Explanation

Put a two under the input a(0)

({}<(()())>) 

While the input is greater than zero subtract one from the input and...

{
({}[()]

Silently...

<

Put one on the other stack to act as a catalyst for multiplication <>(())<>

While the stack is non-empty

 ([])
 {
  {}

Move the top of the list over and copy

  <>({}<<>(({}<>))><>)

Multiply the catalyst by the copy

  <>({<({}[()])><>({})<>}{})<>{}
  ([])
 }
 {}

Add one

 <>({}())

Move the sequence back to the proper stack

 ([])
 {
 {}
 ({}<>)<>
 ([])
 }
 {}
 <>
>)
}{}

Remove all but the bottom item (i.e. the last number created)

([][()])
{
{}
{}
([][()])
}
{}

Answer 12

C, 32 bytes

f(n){return--n?f(n)*~-f(n)+1:2;}

Uses 1-based indexing. Test it on Ideone.

Answer 13

Actually, 9 bytes

2@`rΣτu`n

Try it online!

Uses this relationship instead:

which is derived from this relationship modified from that provided in the sequence:

a(n+1) = a(n) * (a(n) - 1) + 1.

Answer 14

R, 44 42 41 bytes

2 bytes save thanks to JDL

1 byte save thanks to user5957401

f=function(n)ifelse(n,(a=f(n-1))^2-a+1,2)

Answer 15

Python, 38 36 bytes

2 bytes thanks to Dennis.

f=lambda n:0**n*2or~-f(n-1)*f(n-1)+1

Ideone it!

Uses this relationship modified from that provided in the sequence instead:

a(n+1) = a(n) * (a(n) - 1) + 1

Explanation

0**n*2 returns 2 when n=0 and 0 otherwise, because 0**0 is defined to be 1 in Python.

Answer 16

K (oK), 12 bytes

{2|1+*/o'!x}

Try it online!

Blatantly stolen from Port of Adám's answer.

Prints up to the first 11 elements of the sequence, after which the numbers become too big to handle.

Thanks @ngn for 6 bytes!

How:

{2|1+*/o'!x} # Main function, argument x.
       o'!x  # recursively do (o) for each (') of [0..x-1] (!x)
   1+*/      # 1 + the product of the list
 2|          # then return the maximum between that product and 2.

Answer 17

Jelly, 7 bytes

²_’
2Ç¡

Try it online!

Uses this relationship provided in the sequence instead: a(n+1) = a(n)^2 - a(n) + 1

Explanation

2Ç¡   Main chain, argument in input

2     Start with 2
  ¡   Repeat as many times as the input:
 Ç        the helper link.


²_’   Helper link, argument: z
²     z²
  ’   z - 1
 _    subtraction, yielding z² - (z-1) = z² - z + 1

Answer 18

Cheddar, 26 bytes

n g->n?g(n-=1)**2-g(n)+1:2

Try it online!

Pretty idiomatic.

Explanation

n g ->    // Input n, g is this function
  n ?     // if n is > 1
    g(n-=1)**2-g(n)+1   // Do equation specified in OEIS
  : 2     // if n == 0 return 2

Answer 19

CJam, 10 bytes

2{_(*)}ri*

Uses 0-based indexing. Try it online!

Answer 20

05AB1E, 7 bytes

2sFD<*>

Explained

Uses zero-based indexing.

2         # push 2 (initialization for n=0)
 sF       # input nr of times do
   D<*    # x(x-1)
      >   # add 1

Try it online!

Answer 21

R, 50 46 44 bytes

    n=scan();v=2;if(n)for(i in 1:n){v=v^2-v+1};v

Rather than tracking the whole sequence, we just keep track of the product, which follows the given quadratic update rule as long as n>1 n>0. (This sequence uses the "start at one zero" convention)

Using the start at zero convention saves a couple of bytes since we can use if(n) rather than if(n>1)

Answer 22

Jellyfish, 13 bytes

p
\Ai
&(*
><2

Try it online!

Explanation

Let's start from the bottom up:

(*
<

This is a hook, which defines a function f(x) = (x-1)*x.

&(*
><

This composes the previous hook with the increment function so it gives us a function g(x) = (x-1)*x+1.

\Ai
&(*
><

Finally, this generates a function h which is an iteration of the previous function g, as many times as given by the integer input.

\Ai
&(*
><2

And finally, we apply this iteration to the initial value 2. The p at the top just prints the result.

Alternative (also 13 bytes)

p
>
\Ai
(*
>1

This defers the increment until the very end.

Answer 23

Mathematica, 19 bytes

Nest[#^2-#+1&,2,#]&

---

Or 21 bytes:

Array[#0,#,0,1+1##&]&

Answer 24

Prolog, 49 bytes

a(0,2).
a(N,R):-N>0,M is N-1,a(M,T),R is T*T-T+1.

Answer 25

SILOS 201 bytes

readIO 
def : lbl
set 128 2
set 129 3
j = i
if j z
print 2
GOTO e
:z
j - 1
if j Z
print 3
GOTO e
:Z
i - 1
:a
a = 127
b = 1
c = 1
:b
b * c
a + 1
c = get a
if c b
b + 1
set a b
i - 1
if i a
printInt b
:e

Feel free to try it online!

Answer 26

Red, ^{55 49 45} 39 bytes

func[n][x: 2 loop n[x: x * x - x + 1]x]

Try it online!

• -6 thanks to Galen Ivanov
• -4 thanks to Pedro Maimere
• -6 thanks to 9214

This is the first real thing I wrote in Red. You have been warned.

Answer 27

C, 46 bytes

s(n,p,r){for(p=r=2;n-->0;p*=r)r=p+1;return r;}

Ideone it!

Uses p as the temporary storage of the product.

Basically, I defined two sequences p(n) and r(n), where r(n)=p(n-1)+1 and p(n)=p(n-1)*r(n).

r(n) is the required sequence.

Answer 28

C, 43, 34, 33 bytes

1-indexed:

F(n){return--n?n=F(n),n*n-n+1:2;}

Test main:

int main() {
  printf("%d\n", F(1));
  printf("%d\n", F(2));
  printf("%d\n", F(3));
  printf("%d\n", F(4));
  printf("%d\n", F(5));
}

Answer 29

Brachylog, 13 bytes

0,2|-:0&-y+*+

Try it online!

Uses this relationship instead:

which is derived from this relationship modified from that provided in the sequence:

a(n+1) = a(n) * (a(n) - 1) + 1.

Answer 30

Labyrinth, 18 bytes

Credits to Sp3000 who found the same solution independently.

?
#
)}"{!@
* (
(:{

Try it online!